2020 Spring Updates
I became a PhD candidate last Spring and I’m about to start my 5th year here at Cornell University. I started working on the mechanics of laboratory earthquakes at Cornell since 2016. Co-advised by Prof. Dave Kammer and Prof. Greg McLaskey, I use theoretical approaches, e.g., solid mechanics and fracture mechanics, and numerical models, e.g., finite element method and spectral boundary integral method, to understand the mechanics of laboratory-generated earthquakes.
A few weeks before I arrived at Cornell, Prof. Greg McLaskey, Bill and coworkers finished building the 3-m laboratory earthquake apparatus. Hosting two blocks of 3-meter long granite slabs, this apparatus generates up to M -2.5 earthquakes on demand. More importantly, it generates sequences of contained ruptures, which only part of the simulated fault slipped.
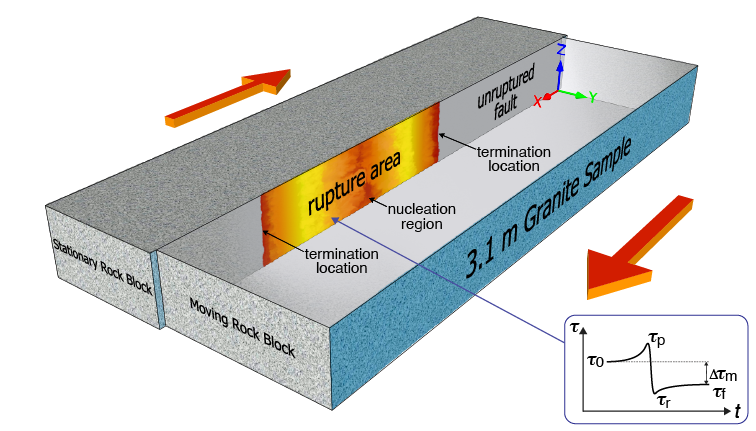
With these laboratory-generated earthquake ruptures that spontaneously arrest before breaking through the whole fault, we concluded that fracture mechanics governs the termination of these frictional ruptures. Without heterogeneities in material properties and geometry, the contained ruptures stopped because it runs out of energy instead of encountering a barrier. This was never achieved before on a flat and dry granite interface and it is more closely resemble the mechanics of natural earthquakes in which the heterogeneity in initial stress plays a more important role in rupture termination. From this experiment we are certain that the exploration of complexity of earthquake ruptures requires a fault that is long enough.
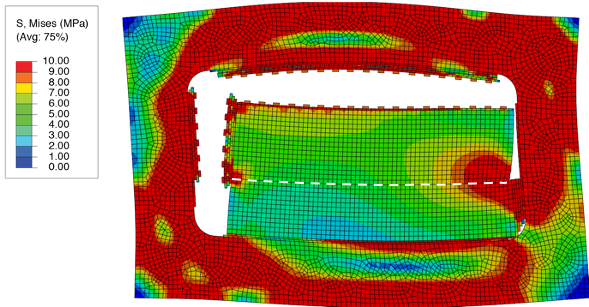
Currently, I’m working on a novel experiment on the quarter-scale apparatus with PMMA (acrylic or plexiglass) samples sandwiching a thin layer of fault gouge. The rate-and-state friction parameters of the fault gouge was meticulously measured in Prof. Chris Marone’s lab at Penn State University. The simulated fault behaves like a ~10x longer rock fault because the surrounding material is ~10x more compliant than rock. With realistic friction and a long fault, we observed interesting behaviors that could link to delayed-triggering and aftershocks that have been observed in natural faults.
We take the friction parameters measured with cm-scaled samples and put it in numerical simulations that has been widely-used for reproducing km-scale fault behaviors to reproduce our m-scale experiments. The numerical simulation is based on spectral boundary integral method first implemented by Prof. Dave Kammer then parallelized and extended by myself and my colleague Gabriele Albertini. Our experiment’s unique length-scale sits right in between those two widely-explored length scales. It is well-suited for validating the application of cm-scale friction laws on km-scale behaviors, and exploring scaling relations and sensitivities of friction parameters.